Gas Law Intro
Gas Laws
We went through a lot of derivations, but the take-home lessons are really not that complicated. There are three basic rules relating to the volume of a gas, all of which are intuitive.
- If you add more molecules (or moles, so that they are easier to count) to a balloon, the volume increases. If you remove molecules, the volume decreases.
- If you raise the temperature of a gas in a balloon, the volume will increase. If you decrease the temperature, the volume will decrease.
- If you increase the pressure on a balloon, the volume will decrease. If you decrease the pressure on the outside of a balloon, its volume will increase.
Volume versus moles
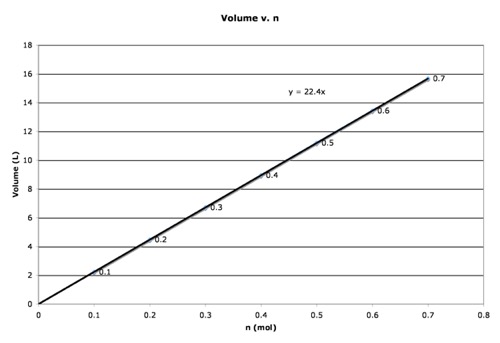
Increasing the number of moles of gas will increase the volume. In fact, if you double the number of moles, the volume will double. You can plot this on a graph of Volume (on Y) versus “n” (number of moles, on X), you get a straight line. This is a simple process. Put in some air, measure the volume. Then put in more, measure the new volume. You then plot the volume (in liters) at each number of moles. The slope of that line is in units of L/mol and is a proportionality constant relating volume to moles. The equation is V = k(n), where k is the slope of the line. A plot is included below. The number (22.4L/mol) is what the slope of the line would be for a gas at 1.00 atmosphere of pressure and 273K. You may note from that statement that we would have a different slope if we did the same experiment at a different temperature or pressure. This equation works only if temperature and pressure are constant. At other temperatures or pressures, you would have different equations.
Volume versus Temperature
If you increase the temperature, the volume also should increase. If we use the absolute scale, we get a graph that looks just like the one we got for volume versus “n.” In this case, the line would be V = k2*T where k2 is the slope of the line in Liters/Kelvin (it’s critical that we use Kelvin for this application). If you use Celsius, you would get the following graph
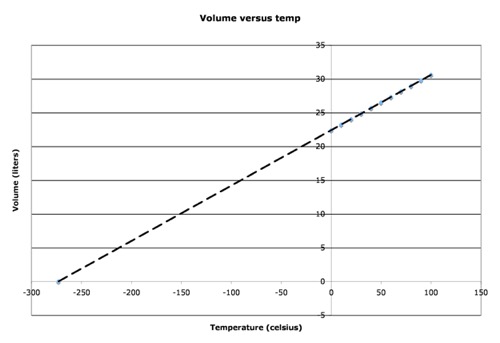
You may notice that there is a point at which the volume is predicted to go to zero. We really don’t expect that to happen, of course. Sooner or later, we would expect that the gas would condense and become a liquid, or even a solid, at which point the volume would not decrease much more. However, that point at which the volume is predicted to go to zero is -273.15 C, which we define as “absolute zero, or 0.0 Kelvin (0.0 K). If we re-plot the same data using the Kelvin scale (just add 273 to degrees celsius), we get the following plot:
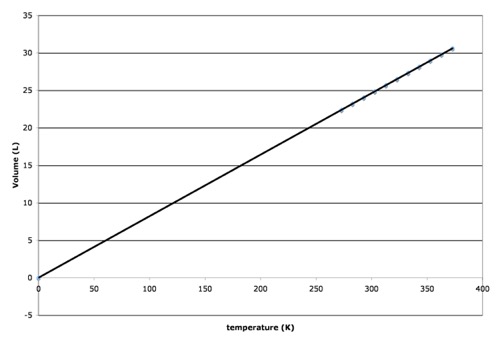
Using Kelvin, you have no negative temperature values. Also, we get a straightforward relationship that if we double the temperature (in K), we double the volume. Once again, we get a straight line with a slope in units of Liters/Kelvin (liters per Kelvin). That slope is the proportionality constant. Again, this line is derived for a particular number of moles and particular pressure.
Volume versus Pressure
If you increase the pressure, in this case, the volume will decrease, while volume will increase if the pressure is decreased. This is known as an “inverse relationship.” So, to get a straight line for this plot, you have to plot Volume versus 1/P.
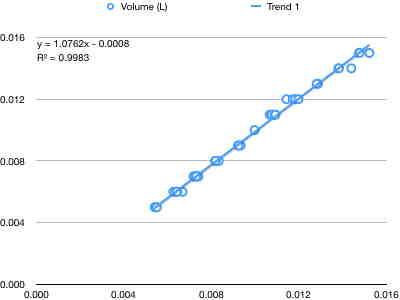
You can the equation V=k3*1/P, which can also be written as P*V =k3, where k3 is the slope of the line and has units of Liter * atmospheres (or liters x whatever unit we use for pressure). All three of these “special” gas laws work only if the other two quantities are held constant.
In other words, the line for volume being proportional 1/P assumes that the temperature and number of moles are constant.
Ideal Gas Law
It would be great if we could derive a single equation that relates all of the parameters. That turns out to be pretty easy: you multiply the three equations and simplify the result. In the end, you get PV=nRT The ideal gas law. P is “pressure (usually in atmosphere in this class), V is volume (always in Liters in this class), ”n“ is number of moles and T is temperature ALWAYS in Kelvin). R is the Ideal Gas constant. The units of R, for us, will usually be liter*atmospheres/mol*Kelvin. In those units, the value is 0.08206 L*atm/mol*K. Using this equation is really quite simple. If I give you any 3 of the unknowns, you can solve for the fourth. For example, if I tell you that pressure is 1.2 atm, temp is 278K and volume is 22.0L, you can calculate how many moles of gas there are in the balloon. As you would for any equation, you just rearrange the equation to get the unknown thing on one side, and leave all the known parameters on the other. Then plug in the values.
Using the equations:
Using this equation is really quite simple. If I give you any 3 of the unknowns, you can solve for the fourth. For example, if I tell you that pressure is 1.2 atm, temp is 278K and volume is 22.0L, you can calculate how many moles of gas there are in the balloon.
As you would for any equation, you just rearrange the equation to get the unknown thing on one side, and leave all the known parameters on the other. Then plug in the values. so, in this case, you are solving for “n” and the equation is which would be.
Notice that the units cancel properly.
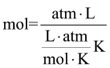
Special Laws
There are also times in which I will not give you enough of the unknowns for you to solve for the last one. Instead I will ask you to tell me the change in one of the parameters given a change in some others. An example would be if I had a 0.57L balloon that was at 285K and I increased the temperature to 305K, what would be the new volume? Once again, the approach would be to rearrange the equation to get all of the parameters that are changing (in this case volume and temp) on one side and the ones that are not changing on the other. So, PV=nRT becomes:
At the first condition, the value is:
But, the values of n, R and P are all constant, so the value of nR/P has to be constant also. That means, that for any temperature and volume, the quotient of V/T has to be constant unless . At the new temperature, 305K, V/305=0.002. You just solve for V.
If you want to skip a step or two, you just set the two conditions equal to each other. That is, you don't even have to know that V/T=.002. Even if you didn't know the pressure and couldn't solve for the value, you would still know that V/T would be constant, as long as P or n do not change. So, as long as that is true:
Just solve for V2.
You can do the same for other "special" cases. Suppose I am holding temperature and number of moles constant, but changing pressure. Let's say, I put a sealed balloon in an oven (code phrases: sealed means "n" is constant…"rigid" means "V" is constant..which doesn't apply here).
You have 0.45L of volume in the balloon at 1.0 atm. Assuming temperature is constant, what is the new volume if I increase the pressure in the room to 1.8atm?
Here, we know n, R and T are constant. PV is changing. So, even though I don't know the temperature and therefore cannot solve explicitly for "n," I do know that P times V will remain constant, just as I showed in the demo today.
So, you can write P1V1=P2V2 and solve for the unknown.
In this case, PV=1.0x0.45=1.8xV2. V2=0.25L
